CBSE Class 12 - Maths - Introduction to Matrices Part -1 (Questions and Answers)(#class12Maths)(#matrices)(#eduvictors)

CBSE Class 12 - Maths - Introduction to Matrices (Questions and Answers)

Part-1

Q1: Name the mathematician, was the first person to introduce the concept of a matrix? Define matrix.

Answer: Arthur Cayley in 1857

A matrix is an ordered rectangular array of numbers or functions. The numbers or functions are called the elements or the entries of the matrix.

Q2: Give examples of domains where matrices based applications are widely used.

Answer: Matrices is one of the most important and powerful tools in mathematics which has found its way into various disciplines like Engineering, Economics, Statistics, Physics, Chemistry, Commerce etc.

Q3: Fill in the blanks with suitable words.

The numbers which form a matrix are called entries or _______ of the matrix. The horizontal lines in a matrix are called _____ and the vertical lines are called ________ of the matrix.

Answer:  elements, rows, columns

Q4: What are the different symbol notations are used to represent matrices?

Answer: A matrix may be represented by the symbol [ ] or ( ) or ||  ||.

Class 12 Syllabus (2021-22) Matrix Chapter:

Concept, notation, order, equality, types of matrices, zero and identity matrix, transpose of a matrix, symmetric and skew symmetric matrices. Operation on matrices: Addition and multiplication and multiplication with a scalar. Simple properties of addition, multiplication and scalar multiplication. Non-commutativity of multiplication of matrices, Invertible matrices; (Here all matrices will have real entries).

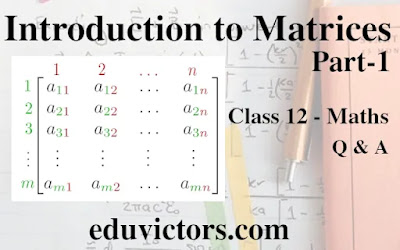
Q5: Represent a matrix A of order m x n in which each element $a_{ij}$, where i represents row element and j represents column element.

Answer:

$\begin{bmatrix} a_{11} &a_{12}  &...  &a_{1n}\\ a_{21} &a_{22}  &...  &a_{2n}\\ a_{31} &a_{32}  &...  &a_{3n}\\  &  &  & \\ a_{m1} &a_{m2}  &...  &a_{mn}\\ \end{bmatrix}$

Q6: What is the order of a matrix?

Answer: A matrix which has m rows and n columns is called a matrix of order m × n, and its represented by $A_{m \times n}$  or $A = [a_{i \times j}]_{m \times n}$.

Obviously, a matrix of order m × n contains mn elements. Every row of such a matrix contains n elements and every column contains m elements.

Q7: For the given matrix:

$\begin{bmatrix}3 &7  &-2 \\ 0 &1  &9 \end{bmatrix}$

Identify

(a) No. of rows

(b) No. of columns

(c) Order of matrix

(d) No. of elements

(e) Value of $a_{21}$

Answer:

(a) No. of rows = 2

(b) No. of columns = 3

(c) Order of matrix = 2 × 3

(d) No. of elements = 6

(e) Value of $a_{21}$ = 0

Q8: If a matrix has 12 elements, what are the possible orders it can have?

Answer: If a matrix has nm elements, then its order is m × n or n × m.

Here mn= 12 = (1)(12) =(2)(6) = (3)(4).

The possible orders of the matrix are: 1 × 12, 12 × 1, 2 × 6, 6 × 2, 3 × 4, 4 × 3.

Q9: If a matrix has 7 elements, what are the possible orders it can have?

Answer: 

If mn = 7 = (1)(7), then the possible orders of the matrices are 1 × 7, 7 × 1.

Q10: Construct a 3 × 2 matrix whose elements are given by $a_{ij}$ = i + 2j

Answer: A 3 × 2 matrix has 3 rows and 2 columns. 

Thus $a_{ij}$  = (i+2j) for i= 1,2,3 and j= 1,2. 

$a_{11}$ = (1 + 2 × 1) = 3; 

$a_{12}$ = (1+2 × 2) = 5;

$a_{21}$ = (2+2 × 1) = 4; 

$a_{22}$ = (2+2 × 2) = 6; 

$a_{31}$ = (3 + 2 × 1) = 5; 

$a_{32}$ = (3 + 2 × 2) = 7.

Hence, A= $\begin{bmatrix}3 &5 \\ 4 &6 \\ 5 &7 \end{bmatrix}$

👉See Also:

Special Mathematical Constants

Types of Vectors

Linear Programming (LPP) - Key Points