Class 11 - Maths - Trigonometric Functions Notes, Solved Problems and NCERT Exercise 3.2 Solutions #eduvictors #class11Maths #Trigonometry

Class 11 - Maths - Trigonometric Functions Notes, Solved Problems and NCERT Exercise 3.2 Solutions

Q1. What are quadrantal angles?

Answer: All angles which are integral multiples of π/2 are called quadrantal angles.

Therefore, for quadrantal angles, we have

cos 0° = 1,

sin 0° = 0,

cos $\frac{π}{2}$ = 0 

sin $\frac{π}{2}$ = 1

cos π = − 1 

sin π = 0

cos $\frac{3π}{2}$ = 0 

sin $\frac{3π}{2}$ = -1

cos 2π = 1 

sin 2π = 0

Trigonometric Functions

sin x = 0 implies x = nπ, where n is any integer

cos x = 0 implies x = (2n + 1) $\frac{π}{2}$, where n is any integer

Other Trigonometric Functions are:

cosec x = $\frac{1}{sin x}$, x ≠ nπ, where n is an integer

sec x  = $\frac{1}{cos x}$, x ≠ (2n + 1)$\frac{π}{2}$, where n is any integer

tan x = $\frac{sin x}{cos x}$, x ≠ (2n + 1)$\frac{π}{2}$, where n is any integer

cot x = $\frac{cos x}{sin x}$, ≠ nπ, where n is any integer

Trignometry Identies:

sin²θ + cos²θ = 1

sec²θ - tan²θ = 1

cosec²θ - cot²θ = 1

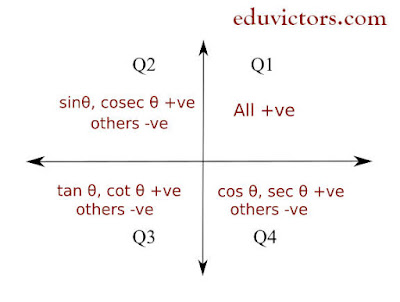
 Trigonometric ratios of various angles:

(sin θ)(cosec θ) = 1, θ ≠ nπ, where n ∈ Z

(cos θ)(sec θ) = 1, θ ≠

Q2. Find the values of the other five trigonometric functions if , cos x = $\frac{-1}{2}$ lies in the third quadrant.

Answer:  cos x = $\frac{-1}{2}$

∴ sec x = $\frac{1}{cos x} = \frac{1}{\frac{-1}{2}} $

∴ sec x = -2

Since, sin²θ + cos²θ = 1

∴ sin²x = 1 - cos²x 

sin²x = 1 - $(\frac{-1}{2})^{2}$

sin²x = 1 - $\frac{1}{4}$

sin²x = $\frac{3}{4}$

sin x = ±$\frac{\sqrt{3}}{2}$

Since x lies in the 3rd quadrant, the value of sin x will be negative

∴ sin x = -$\frac{\sqrt{3}}{2}$

cosec x = $\frac{1}{sin x}$

cosex x = $\frac{1}{-\frac{\sqrt{3}}{2}}$

cosec x = $\frac{2}{\sqrt{3}}$

tan x = $\frac{sin x }{cos x}$

tan x = $\frac{\frac{\sqrt{3}}{2}}{\frac{-1}{2}}$

tan x = $\sqrt{3}$

cot x = $\frac{1}{tan x}$

cot x = $\frac{1}{\sqrt{3}}$

Q3. Find the values of the other five trigonometric functions if sin x = $\frac{3}{5}$, x lies in the second quadrant.

Answer: sin x = $\frac{3}{5}$ 

cosec x = $\frac{1}{sin x} =\frac{1}{\frac{3}{5}}$

cosec x = $\frac{5}{3}$ 

Since, sin²x + cos²x = 1

cos²x  = 1 - sin²x 

cos²x  = 1 - $(\frac{3}{5})^2$

cos²x  = 1 - $\frac{9}{25} = \frac{16}{25}$

cos x = ±$\frac{\sqrt{4}}{5}$

Since x lies in the 2nd quadrant, the value of cos x will be negative. 

∴ cos x = -$\frac{\sqrt{4}}{5}$

sec x = $\frac{1}{cos x} = \frac{-5}{4}$

tan x = $\frac{sin x}{cos x} = \frac{-3}{4}$

cot x = $\frac{1}{tan x} = \frac{-4}{5}$

Q4. If $sin \theta = \frac{a- 4}{2}$ then a lies in ?

Answer: Since -1 ≤ sin θ ≤ 1

Thus we have,

-1 ≤ $\frac{a-4}{2}$ ≤ 1

⇒ -2 ≤ a - 4 ≤ 2

⇒ 2 ≤ a ≤ 6

⇒ a ∈ [2, 6]

Important points you should know:

(a) If A + B = 90° or 270°, then

(i) sin²A + sin²B = 1

(ii) cos²A + cos²B = 1

(iii) tan A . tan B = 1

(iv) cot A . cot B = 1

(b) If A + B = 180°, then

(i) cos A + cos B = 0

(ii) sin A - sin B = 0

(iii) tan A + tan B = 0

(c) If A + B = 360°, then

(i) sin A + sin B = 0

(ii) cos A - cos B = 0

(iii) tan A + tan B = 0

Q5. sin²35 + sin²55 =?

Answer: Since 35° + 55° = 90°

∴ sin²35 + sin²55 = 1

Q6. Find the value of the trigonometric function sin 765°

Answer: We know that the values of sin x repeat after an interval of 2π or 360°. 

∴ sin 765° = sin(2 × 360° + 45°) = sin 45° = $\frac{1}{\sqrt{2}}$

Q7. Find the value of the trigonometric function cosec(–1410°)

Answer: cosec(–1410°) = –cosec(1410°)

We know that the values of cosec x repeat after an interval of 2π or 360°.

∴ -cosec(-1410°) = -cosec (4 × 360° - 30°)

= cosec(30°)

= 2

Q8. Find the value of the trigonometric function $\tan{\frac{19\pi}{3}}$

Answer: We know that the values of tan x repeat after an interval of π or 180°

Given $\tan{\frac{19\pi}{3}} = \tan{6\pi + \frac{\pi}{3}}$

 = $\tan{\frac{\pi}{3}}$

 = tan 60°

 = $\sqrt{3}$

 

👉See Also:

Ch2: Relations and Functions (1 Mark Q & A) Part-1
Ch2: Cartesian Product of Two Sets (Important Points)
Ch2: Relations - Domain, Range and Co-Domain (Solved Problems)
Ch3: Trigonometric Functions + NCERT Ex 3.1
Ch5: Complex Numbers (Part 1) - Solved Problems
Ch 13 Limits and Derivatives (Q & A ) Part -1